Cheng He, Minghui Lu, and Yanfeng Chen’ research groups, working with Haijun Zhang’s research group of School of Physics, theoretically designed and experimentally synthesized a special Hybrid acoustic topological insulator(TI) in three dimensions(3D). Different from the surface Dirac cones of normal TIs where dispersion is linear in all directions, the surface states of this acoustic TI posses directional dispersion:linear in one direction and quadratic in another direction. This work demonstrates the ability to design, tune, and manipulate acoustic topological surface states through artificial microstructure, which may pave the way for future required design of acoustic topological devices or even acoustic topological band engineering. The manuscript titled as Hybrid Acoustic Topological Insulator in Three Dimensions is published online on Physical Review Letters on 6th November, 2019.[Phys. Rev. Lett.123, 195503 (2019)]
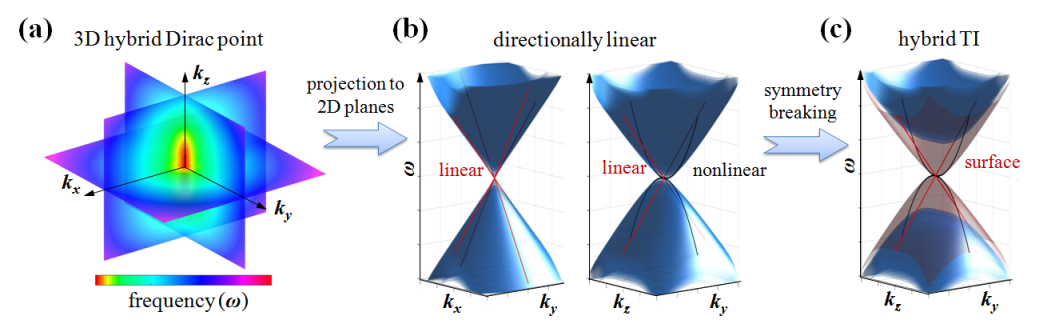
Figure 1.(a) Schematic of a 3D hybrid Dirac point for bulk bands.(b) Projection hybrid Dirac cone to the kxy and kzy planes,where dispersion along kx or ky direction is linear and dispersion along kz direction is quadratic.(c) Directional dispersion of 2D topological surface states
Research on topological states of electronic systems is one of the front directions of condensed matter physics. Over the past decade, the researchers have extended topological design into photonics, acoustics, mechanics, and many other classical wave systems. One well-known case is the optical or acoustic TI states, which exploit the symmetry of artificial microstructure to topologically clip the band of phononic or photonic crystals. By doing so a pair of optic and acoustic waves only travelling along the edges of the material could be realized. Robust transport brought by spin-orbit coupling is also supported. Progress already made mainly lies in two-dimensional(2D) systems, where various kinds of 2D optical/acoustic topological states have been explored. Their one-dimensional(1D) topological edges have shown prospects with respect to strange phenomenon and devices for topological protection, such as backscattering suppression/defect immunity/anti radiation-loss optic or acoustic waveguides, topologically directional antennas, arbitrary shaped high efficiency topological lasers and so on. Recently, optic/acoustic topological states in 3D systems have draw a lot of attention. Its potential advantage is that it could provide 2D topological planes to manipulate waves and realize some topological phenomena and functions that 1D waveguides could not do such as refraction and imaging. The main challenge of realizing airborne 3D acoustic TIs comes from the spinless nature of airborne sound. One solution is to generate the acoustic pseudospins by employing the symmetry of artificial mircrostructures. Although previous studies have shown that 2D acoustic topological states with acoustic pseudospins can be realized, it’s difficult to directly stack them to form 3D acoustic TIs due to the fact that interlayer coupling will destroy the spatial symmetry based pseudospins.
After previous experiments and theoretical research on acoustic/optical topological states [Nat.Phys.12,1124(2016);PNAS113, 4924(2016); Nat.Commun.9, 3072 &.9,4555(2018)], this work developed a new mechanism ,that is, realizing 3D acoustic TI states by means of opening up degeneracy on hybrid acoustic Dirac point. Generally speaking, dispersion on Dirac point or Weyl point in 3D systems is linear in all directions. This paper, however, considers a degeneracy point where dispersion is directionally linear, as is shown in Fig. 1: dispersion is linear along kx and ky direction, but quadratic along kz direction. Through breaking certain symmetry, a topological bulk band gap could be formed by opening up the degeneracy point. Among the gap 2D acoustic topological surface states with directional dispersion is realized.
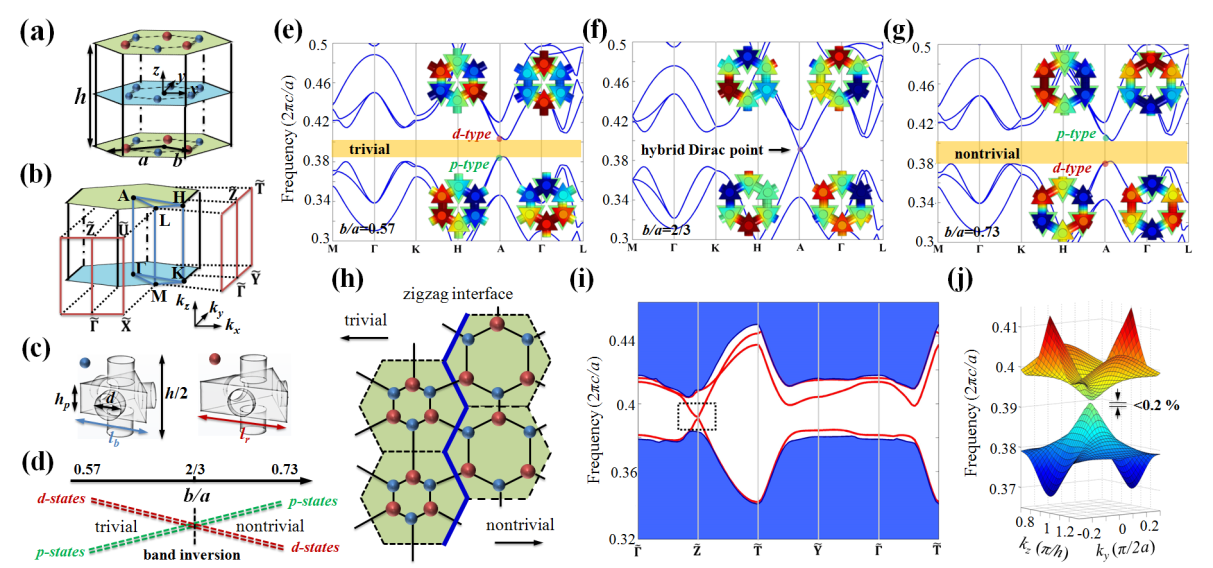
Figure 2.(a) Schematic of acoustic crystal;(b)Schematic of bulk and lateral Brillouin zone;(c) The triangular prism cavities and connecting tubes represent the acoustic atoms and their couplings;
(d) Bands inversion by stretching and compressing lattice;(e)-(g) Bulk band structures at (e) b/a= 0.57, (f)b/a = 2/3, and (g) b/a = 0.73;(h)Schematic of the zigzag interface with topological trivial and nontrivial acoustic crystals;(i) The projected band structures, where the red lines represent the acoustic surface states;(j) conical-like2D acoustic surface states
With respect to material design, this work considered acoustic microcavities and constructed a acoustic crystal by a double-layer honeycomb lattice. One layer is composed of identical acoustic atoms while another layer consists of alternating two different atoms. By stretching and compressing the inner hexagons in the xy plane, we can realize the open-close-open process of full bulk bands in acoustic crystals, namely band inversion. Therefore, nearly gapless 2D topological surface states could be realized on the lateral interface of topological and trivial acoustic crystals. This topological band is realized by opening up the hybrid Dirac degeneracy point (fourfold) on point A in Brillouin zone. Under such circumstances, there exists no coupling between layers in acoustic crystals. The hexagonal structure consisting of identical acoustic atoms guarantees the linear dispersion within kxy plane. With the increasing distance from point A along kz direction, coupling between layers is gradually strengthened, featuring quadratic dispersion. As is shown in Fig. 3, the equifrequency surface calculated by numerical simulation and tight-binding model suggests that surface hybrid Dirac dispersion is endowed with obvious anisotropy. It is worth noting that there is only one surface conical-like cone in the whole Brillouin zone, which may be viewed as a directional special topological phenomenon and a material between strong TI(odd surface Dirac cones) and weak TI(even surface Dirac cones).
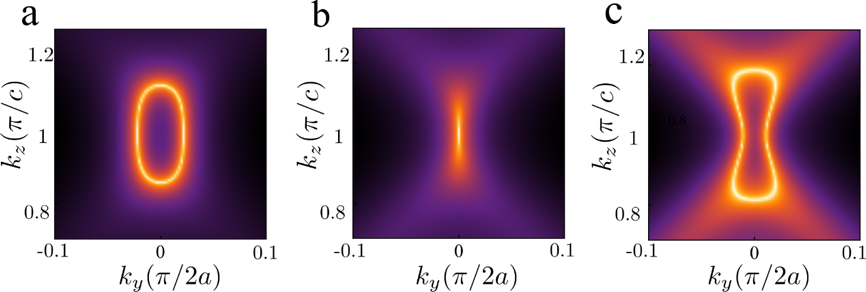
Figure 3. Equifrequency surfaces of surface hybrid Dirac dispersion based on tight-binding model.(a) frequency above hybrid Dirac point;(b) frequency at hybrid Dirac point;(c) frequency below hybrid Dirac point
In experiment, the research group precisely set the structural parameters and prepared the 3D acoustic topological crystal by 3D printing(Fig. 4(a)). And the research group measured directional transmission spectra of 2D acoustic topological surface states of the sample(Fig.4(b-d)) and verified the gapless behaviour along the positive kzdirection. With the increasing incident angle, the band gap of its topological surface states was gradually opened. Besides, the surface acoustic field distributions along the z direction at an open boundary was also measured. The surface dispersion was obtained by the Fourier transformation of the surface acoustic field distributions, thus confirming the existence of conical-like 2D surface dispersion.
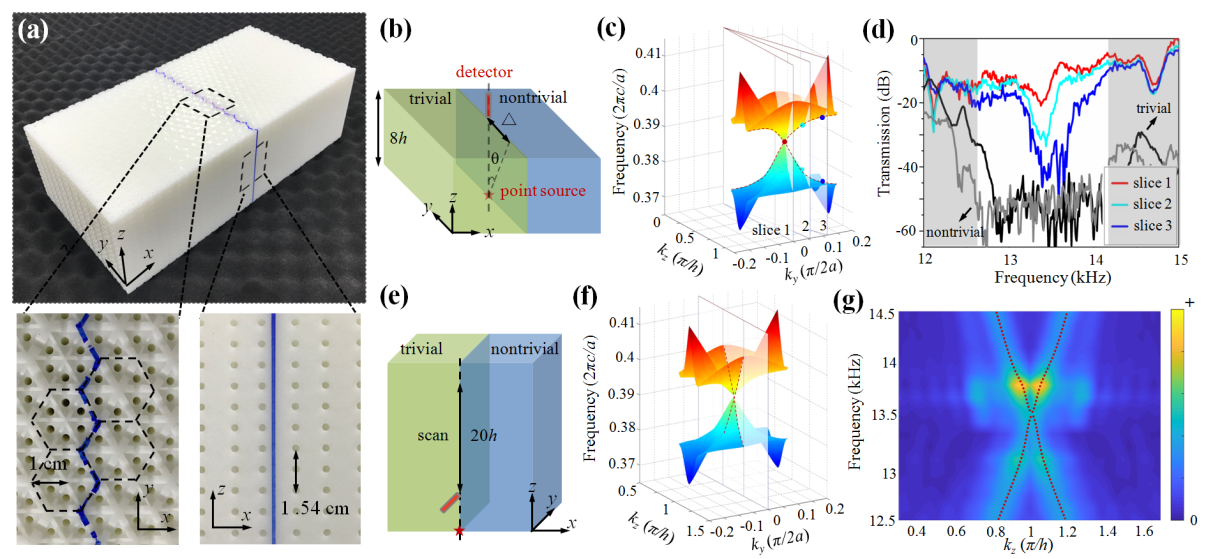
Figure 4.(a) Schematic of acoustic crystal sample.Lower left: magnified top-down view. Lower right: magnified lateral view.(b) Experimental configuration to measure directional transmission spectra of surface states.(c) Three 2D-k slices on conical-like surface dispersion with θ = 0, arctan(0.5a/8h), and arctan(a/8h).(d) Experimentally measured transmission spectra.The surface transmission spectra for slices 1–3 are represented by red, cyan, and blue lines, respectively.
(e)Experimental configuration to map the surface dispersion along the z direction.
(f) A slice at ky= 0.(g) The measured surface dispersion along z direction.
The importance of this work lies in: 1) proposing and realizing brand new topological surface states with directional dispersion, which is not reported yet in other systems. This means acoustic topological systems not only can serve as a platform where known topological materials get verified, but also be used to research and explore unknown topological phenomena, which can deepen people’s understanding of the very essence of topology. 2) the manipulation of acoustic topological surface dispersion hopefully could be utilized in fruitful applications such as directionally slow sound, robust acoustic surface sensing and imaging based on the 2D topological interfaces.
This work is the result of close combination of theoretical design, model construction, material preparation, and precise measurement. Cheng He, Siyuan Yu of College of Engineering and Applied Science and Huaiqiang Wang of School of Physics are joint first authors of the paper. Haijun Zhang, Minghui Lu and Yanfeng Chen are co-corresponding authors of the paper. Hao Ge, Jiawei Ruan also contribute a lot to the paper. This work is supported by the National Key Research and Development Program of China, the National Natural Science Foundation of China and many other funding projects.

